We present the model published in [Mat15a] .
The differential cross section for $\gamma p\to\pi^0 p$ is computed with Regge amplitudes in the domain $E_\gamma\ge 4$ GeV and $0.01\le |t| \le 3$ (in GeV$^2$).
The formulas can be extrapolated outside these intervals.
We use the CGLN invariant amplitudes $A_i$ defined in [Chew57a].
See the section Formalism for the definition of the variables.
The fitting procedure is detailed in [Mat15a] . We report here only the main features of the model.

Formalism

The differential cross section is a function of 2 variables. The first is the beam energy in the laboratory frame $E_\gamma$ (in GeV) or the total energy squared $s$ (in GeV$^2$). The second is the cosine of the scattering angle in the rest frame $\cos\theta$ or the momentum transfered squared $t$ (in GeV$^2$).
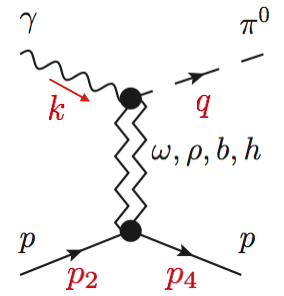
The momenta of the particles are $k$ (photon), $q$ (pion), $p_2$ (target) and $p_4$ (recoil). The pion mass is $\mu$ and the proton mass is $M$. The Mandelstam variables, $s=(k+p_2)^2$, $t=(k-q)^2$, $u=(k-p_4)^2$ are related through $s+t+u=2M^2+\mu^2$.

The differential cross section is expressed in term of the parity conserving helicity invariant amplitudes in the $t-$channel $F_i$ \begin{align}\nonumber \frac{d\sigma}{dt} = \frac{389.4}{64\pi} \frac{k_t^2}{4 M^2 E_\gamma^2} \left[ 2\sin^2\theta_t \left( t |F_1|^2 +4 p_t^2 |F_2|^2 \right) + (1-\cos\theta_t)^2 \left| F_3+2\sqrt{t} p_t F_4\right|^2 + (1+\cos\theta_t)^2 \left| F_3-2\sqrt{t} p_t F_4\right|^2 \right] \end{align} The differential cross section is expressed in $\mu$b/GeV$^2$. We used $(\hbar c)^2=0.3894$ mb.GeV$^2$.
The $t-$channel is the rest frame of the process $\gamma \pi^0 \to p\bar p$.
In the $t-$channel, the momenta of the nucleon $p_t$ and the pion $k_t$ and the cosine of the scattering angle are \begin{align} k_t & = \frac{1}{2}\sqrt{t-4M^2}, & q_t&= \frac{t-\mu^2}{2\sqrt{t}}, & \cos\theta_t &= \frac{s-u}{4 p_t k_t}. \end{align}

The invariant amplitudes $F_i$ are related through the CGLN $A_i$ amplitudes [Chew57a] by \begin{align} \label{eq:F1} F_1&= -A_1+2MA_4,& \eta &= +, & CP&=+\\ F_2&= A_1+t A_2 ,& \eta &= -, & CP&=-\\ F_3&= 2M A_1-t A_4,& \eta &= +, & CP&=+\\ \label{eq:F4} F_4 &=A_3& \eta &= -, & CP&=+\\ \end{align} The $F_i$ amplitudes have good quantum numbers of the $t-$channel, the naturality $\eta=P(-1)^J$ and the product $CP$.
$C$ and $P$ are the charge conjugation and the parity. $J$ is the total spin.

Model

The model is based on the exchange of the leading Regge singularities.
Since $C(\gamma\pi^0) = -\gamma\pi^0$, the only quantum numbers allowed in the $t-$channel are \begin{align} \rho &: I^{G\tau\eta}=1^{+-+}, & F_1,F_3\\ \omega &: I^{G\tau\eta}=0^{--+}, & F_1,F_3\\ b &: I^{G\tau\eta}=1^{+--}, & F_2\\ h &: I^{G\tau\eta}=0^{---}, & F_2\\ \end{align} The second column indicates the amplitudes which the exchange contributes to.
$G=C(-1)^I$ is the $G-$parity and $\tau=(-1)^J$ is the signature.
The quantum numbers of the trajectories are denoted by the lowest spin particles.
Our model is insensitive to the isospin. We will refer to vector for the $\rho$ and $\omega$ trajectories and to axial-vector for the $b$ and $h$ trajectories.

The $t$ dependence of the amplitudes is determined by matching the exchange of the lowest particles in the CGLN basis. The amplitudes involve the vector ($\alpha_V$) and axial-vector ($\alpha_A$) poles but also the vector cut ($\alpha_c$) trajectories \begin{align} \label{eq:F1Reg} F_1 &= (-g_1 t+2Mg_4) R(\alpha_V) + (-g^c_1 t+2Mg^c_4) R_c(\alpha_c) ,\\ F_2&= g_2 t R(\alpha_A),\\ F_3&= (2M g_1 - g_4) t R(\alpha_V) + (2M g^c_1 - g^c_4) t R_c(\alpha_c),\\ \label{eq:F4Reg} F_4 &=0. \end{align} The $g_i$ are real parameters, the couplings.
The energy dependence is given by the Regge prescription (with $s_0=1$ GeV$^2$) \begin{align} R(\alpha) & = \frac{\pi}{\Gamma(\alpha)} \frac{1-e^{-i\pi\alpha}}{2\sin \pi \alpha} \left(\frac{s}{s_0}\right)^{\alpha-1}, \\ R_c(\alpha) & = \frac{1}{\log(s/s_0)} \frac{\pi}{\Gamma(\alpha)} \frac{1-e^{-i\pi\alpha}}{2\sin \pi \alpha} \left(\frac{s}{s_0}\right)^{\alpha-1}. \end{align} Finally we use linear Regge trajectories \begin{align} \alpha_V(t) &= \alpha_{V0}+ \alpha_V' t, & \alpha_A(t) &= \alpha_{A0}+ \alpha_A' t, & \alpha_c(t) &= \alpha_{c0}+ \alpha_c' t. \end{align}

We have fitted the 11 parameters. The result is \begin{align} \label{eq:param1} g_1 &= \phantom{+0} 1.24\pm 1.56 \text{ GeV}^{-4}, & \alpha_{V0} & = 0.54 \pm 0.03\\ g_4 &= -6.68\pm 0.80 \text{ GeV}^{-3}, & \alpha_{V}' & = 1.34 \pm 0.08 \text{ GeV}^{-2}\\ g_1^c &=\ \,-2.36 \pm 0.36 \text{ GeV}^{-4}, & \alpha_{c0} & = 0.43 \pm 0.03\\ g_4^c &=\ \, -4.26 \pm 0.16 \text{ GeV}^{-3}, & \alpha^\prime_c &= 0.16\pm0.01 \text{ GeV}^{-2} \\ g_2 &= - 9.74\pm 2.96 \text{ GeV}^{-4}, & \alpha_{A0} & = -0.22 \pm 0.33\\ \label{eq:param5} & & \alpha^\prime_A &= \phantom{-} 1.08\pm 0.21 \text{ GeV}^{-2}. \end{align}

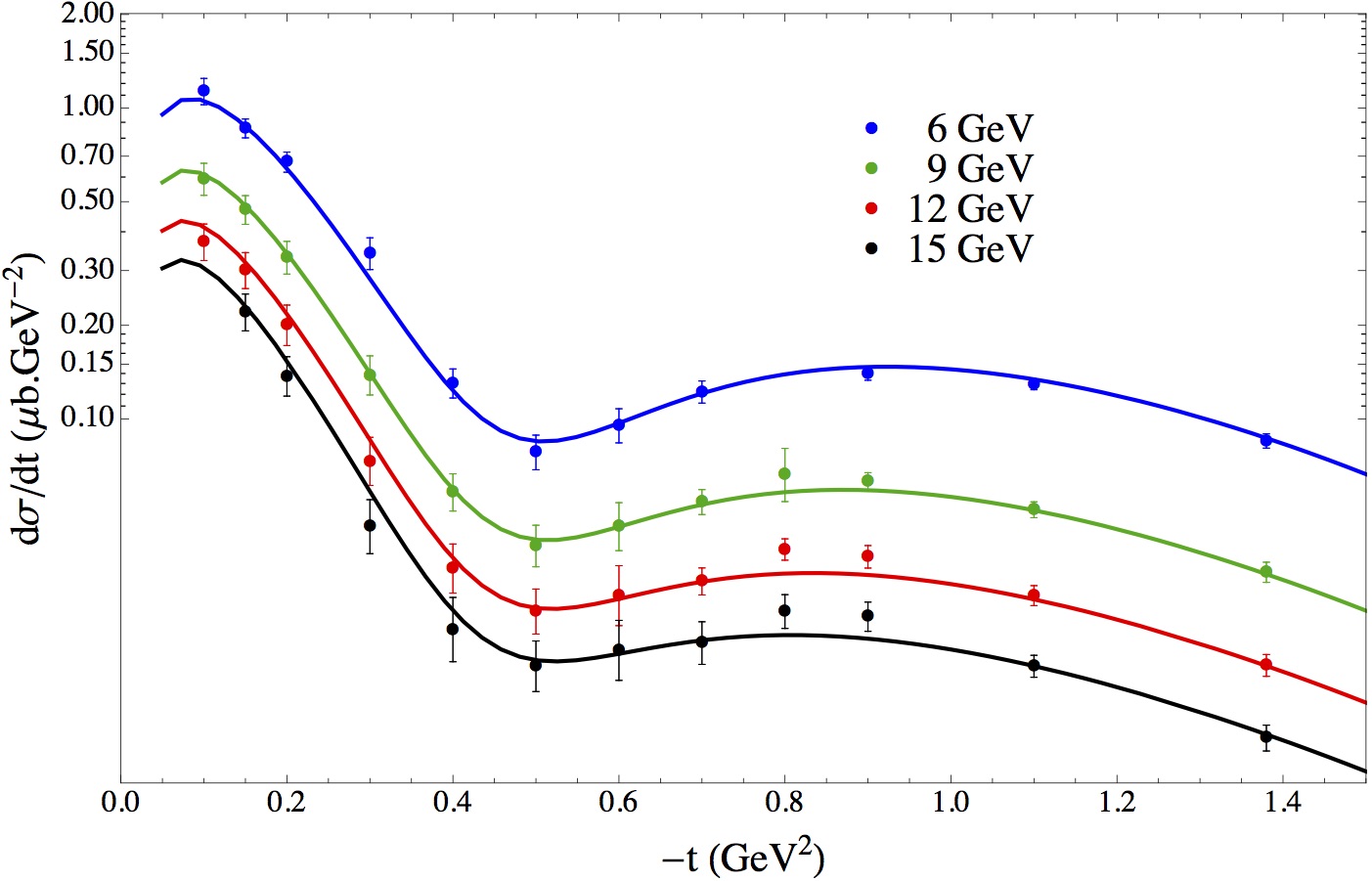
The results is presented below and compared to the data from [And71a].

References

[Chew57a]
G.F. Chew, M.L. Goldberger, F.E. Low and Y. Nambu,
``Relativistic dispersion relation approach to photomeson production,'' Phys. Rev. 106, (1957) 1345.

[And71a]
R. L. Anderson et al.,
``High-energy $\pi^0$ Photoproduction from hydrogen with Unpolarized and Linearly Polarized Photons," Phys. Rev. D4 (1971) 1937.

[Mat15a]
V. Mathieu and A. Szczepaniak,
``Neutral Pion Photoproduction in a Regge Model,'' arXiv:1505.02321 [hep-ph]

Resources

• Publication: [Mat15a]
• Fortran: Fortran file, Input file, Output file
• C/C++: AmpTools class, C/C++ file, AmpTools class header, C/C++ file header.
• Mathematica: notebook , converted in text
• Data: Anderson, All data
• Contact person: Vincent Mathieu
• Last update: November 2015

Run the code